Condorcet Internet Voting Service

I’d like to find out which world map projections people prefer. The poll results will display the “finishing-order” of the projections, the order in which they’re preferred.

The projections will be shown below

In this poll, vote as you would if we were voting on which world map projection should be used in a world atlas, or which world map projection should be adopted for use in National Geographic magazine, for its world maps.

Rank, in order of preference, as many or as few projections as you wish.

If you don’t want to rank all 8 map projections, then just rank the best ones.

You can rank 2 or more projections equal if you wish.

You needn’t include “Acceptability Dividing-Line” in your ranking. But if you want to indicate that a projection is unacceptable, or isn’t good enough for consideration, than you can express that by ranking “Acceptability Dividing Line” somewhere over it.

The rankings will be counted by one of the count-methods offered at this poll's "Results" page. It's known as Maximize Affirmed Majorities (MAM). It's one of the two widely-accepted best ideal majoritarian rank-counts. I consider it the better of those two, because it more often reflects majority wishes, and because its definition and rule are brief, with the most obvious and clear justification and motivation.

The "Results" page of this polling website has a link for more information about MAM, and for voting-system information in general.

(Note: The "Acceptability Dividing Line" is not a feature of MAM, and wouldn't be included in an official political election)

Below this poll-description (which includes images and brief descriptions of all 8 maps), will be the balloting-area.

Some of the projections' construction-explanations are long. That of the August projection is particularly long.

So I want to emphasize that the construction-explanations are included purely as a matter of curiosity, for those who want to hear about it--and are not necessary for judging the merits of the projections or voting on them.

Of course all the information in this poll-description is optional--Look at as much or as little of it as you want to, and base your vote on whatever considerations you want to.

...such as how good the map looks, or how useful it seems. No part of this poll description is essential for your voting choices--what you go by is up to you.

Let me define two important and useful map properties:

(These properties of the individual maps will be stated in the brief map-descriptions that immediately follow the map images)

A map is “equal-area” if all regions are shown in their correct area-proportions. The map’s area-relations are exactly correct.

A map is “conformal” if:

1. At every point on the map, the scale is the same in every direction….2. Small shapes are correct ….3. At every point, directions are perfectly accurate (measured with respect to the latitude and longitude lines).

#2 and #3 result from #1.

Equal-area maps, of course, are useful when it’s desired to compare areas of continents, countries, or other regions.

Conformal maps are useful for most clearly showing the shapes and appearance of regions, showing directions, and measuring or judging distances (because the scale is everywhere the same in all directions).

No map can be both equal-area and conformal.

For those interested in construction, the following 3 azimuthal projections must be defined, because they're the basis of the construction of the equal-area and conformal maps shown below:

Azimuthal maps are circular, point-centered, radial maps.

1. If the radial scale is constant, then the map is Azimuthal Equidistant.

2.If the radial distances from the center are drawn so that, at every point on a radius, the radial scale equals the circumferential scale at that point, then the map is conformal (by the above definition), and is called the Stereographic Projection.

3. If the radial distances from the center are drawn so that, at every point on a radius, the product of the radial and circumferential scales remains constant, then the map is equal-area (as defined above), and is called Azimuthal Equal-Area.

The name of each projection will be written directly above and to the left of the map's image. Below each image will be a description of the projection.

Aitoff:

Introduced 1889.

Construction: An Azimuthal Equidistant map of half the Earth, with its center on the equator, is expanded horizontally, doubling its width.

Advantage: Realistic elliptical Earth portrayal. Though it isn’t equal-area or conformal, it’s very nearly equal-area.

Hammer:

Introduced 1892, based on Aitoff.

Construction: An Azimuthal Equal-Area map of half of the Earth, with its center on the equator, is expanded horizontally, doubling its width.

Equal-area. Elliptical, with nearly as good globe-realism as Aitoff.

Quartic:

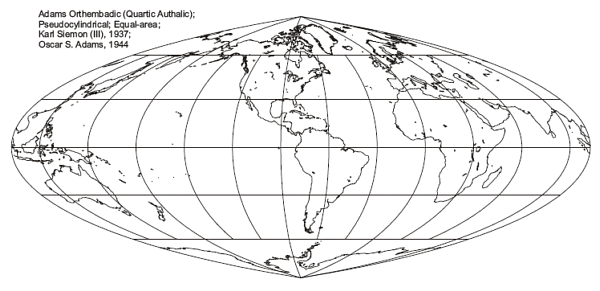
Equal-Area

Introduced 1945

Construction: It’s as if (as was probably the case) someone wanted to make Hammer pseudocylindrical (straight horizontal parallels, each with its own constant uniform scale).

The straight horizontal parallels are spaced as are Hammer’s parallels at Hammer’s central meridian. Each parallel is extended outward from the central meridian far enough so as to make the map equal-area. Then the map is horizontally expanded slightly to optimize shape.

Advantages: Equal-area. Resembles Hammer, but is pseudocylindrical..

Trades some of Hammer’s globe-realism for a pseudocylindrical map's convenience in finding &/or identifying positions.

.

Stereographic

Conformal. Max-scale/Min-scale = 2.

This is the Stereographic in the form of two side-by-side circles. It’s the Stereographic projection interrupted on two opposite meridians.

It was widely published in the 1400s and 1500s, but it goes back to classical or ancient Greece.

Construction: The Stereographic is an “azimuthal” (radial, point-centered) map. Radial distances out from the center geographic-point are drawn so that, at every point on a radius, the radial scale is the same as the circumferential scale at that point on the map. That makes the map conformal, as defined above.

Though the above-described construction principle is sufficient—and such a principle has sometimes used directly to construct a conformal world map—the Stereographic has an easy geometric projective construction technique, known and used since classical or ancient times:

If there is a plane surface tangent to the globe’s surface, and there is a point-source of light on the globe at the point directly opposite to the plane’s point of tangency, then that light-source will project, onto the plane, a Stereographic map of the half of the Earth closest to the plane.

All world maps are interrupted on at least one meridian (the boundary of the map). Most world maps--the ones that are in one piece and don’t have “interrupted” in their name--are interrupted on only one meridian.

Being interrupted on two meridians (instead of one, as are most world maps), reduces the map’s maximum scale variation, its max-scale/min-scale. Conformal maps tend to have more regional magnification, max-scale/min-scale. That regional magnification is reduced by having interruption on 2 meridians instead of 1.

Advntages: Conformal. Relatively low regional magnification, for a conformal world map.

August:

Conformal. Max-scale/Min-scale = 8.

Introduced 1874.

(The August construction-explanation, immediately below, is long. It's only included as a matter of curiosity, for those who want to hear about it. It isn't necessary for your evaluation of August. It isn't necessary for your vote. Your ranking should be based on what projections you like...which ones you regard as interesting, useful, &/or aesthetically-appealing. Feel free to skip the following August construction-explanation if it isn't something that you want to find out about.)

Construction:

August is constructed by the use of Lagrange, which is constructed by the use of Mercator and Stereographic.

So, first, a brief mention of the constructions of Mercator and Lagrange.

Mercator: Similar to what was said above about Stereographic’s construction: Mercator is a conformal map centered on a straight horizontal equator. Distances north or south of the equator are drawn in such a way that, at each point, the north-south scale at that point is equal to the east-west scale at that point. Direct application of that principle was the method by which Mercator was originally constructed. That’s a numerical solution of the differential equation. Later, when the calculus was introduced, Mercator was constructed by formula.

Lagrange:

Construct, at the center of a Mercator map, another Mercator map with half the width of the 1st map. Reverse-map the large Mercator back onto the globe. Now, from the smaller Mercator, you have the entire Earth mapped onto half of the globe. Map that half of the globe with the Stereographic projection.

That gives you the Lagrange projection. …The entire Earth shown conformally in a circle. (not shown here due to space considerations)

Construction of August from Lagrange:

Each point on Lagrange is represented as a complex number (called z), with its “imaginary” portion the point’s horizontal (X) co-ordinate, and its “real” portion the point’s vertical (Y) co-ordinate. By this system, Lagrange’s north pole is 1 + 0i, and its south pole is -1 + 0i.

The west and east ends of the equator are, respectively, 0 - 1i, and 0 + 1i.

The cubic function 3z - z^3 is applied to each point’s complex number. That function gives a new complex number for each point. The resulting new set of complex numbers, and the co-ordinates that each represents, is the August projection picture of the Earth.

When a polynomial function (like the cubic function) is applied to the complex numbers representing a map’s points, then, if the original map was conformal, then the resulting new map will be conformal too.

The cubic function 3z - z^3 was chosen to give August’s polar-cusped shape.

August, like the above-shown Stereographic, is conformal everywhere, including the poles. That’s an accomplishment, for a map interrupted only on one meridian.

That is made possible by August’s polar “cusps”.

Additionally, due to the cusps, August is able to have low polar magnification, small Antarctica, and low max/min scale variation, for a conformal map interrupted on only one meridian.

For comparison, August's max/min scale is 8, while Lagrange's max/min scale is two or three hundred (...because Lagrange has very high magnification right at the poles).

If you think August's Antarctica is large, you should see that of Lagrange. That isn't intended as criticism of Lagrange, but only as an explanation for how August improves on Lagrange, and why August is included here.

Someone could say, “But the Earth isn’t split like that!” No, but every flat world map splits the Earth. Lagrange, like all un-cusped world maps interrupted on only 1 meridian, splits the Earth more than August does. For maps in equatorial-aspect, both boundary-lines leading away from the south poll are the same meridian. On un-cusped flat world maps, interrupted on only one meridian, along those lines, 2 adjacent strips of earth-surface have been split from eachother by 180 degrees, at the pole.

So August is considerably _less_ split than Lagrange, or other un-cusped world maps interrupted on only one meridian. That’s what achieves its conformality everywhere, including at the poles; its lower max/min scale; and its smaller Antarctica.

Briesemeister

Introduced 1953.

Briesemeister is Hammer, from a different point of view. It’s the big-landmass-centered Hammer map.

Advantages: Equal area. It’s centered on the center of the Earth’s largest land-masses. Once the Earth’s continents were all one big landmass, called Pangea. Briesemeister shows how close Eurasia, Africa, and the Americas still are, and the extent to which they can still be regarded as one big landmass with a little split (the Atlantic Ocean).

Robinson:

Neither conformal nor equal-area

Introduced 1963

Intended as a compromise between conformality and equal-area. Some semblance of each, but all of neither.

Scale is infinite along the pole-lines, and so max/min scale is infinite.

NGS Winkel:

Neither conformal nor equal-area

Introduced 1920s

Likewise intended as a compromise between conformality and equal-area. But look at its shapes...

Like Robinson, Winkel has infinite scale along its pole lines, and therefore has infinite max/min scale.

End of poll-description. Vote below by ranking the projections in order of preference: